"In order to design an orthokeratology lens, we first need to assume a target model eye, and then place our orthokeratology lens on the cornea of this model eye.
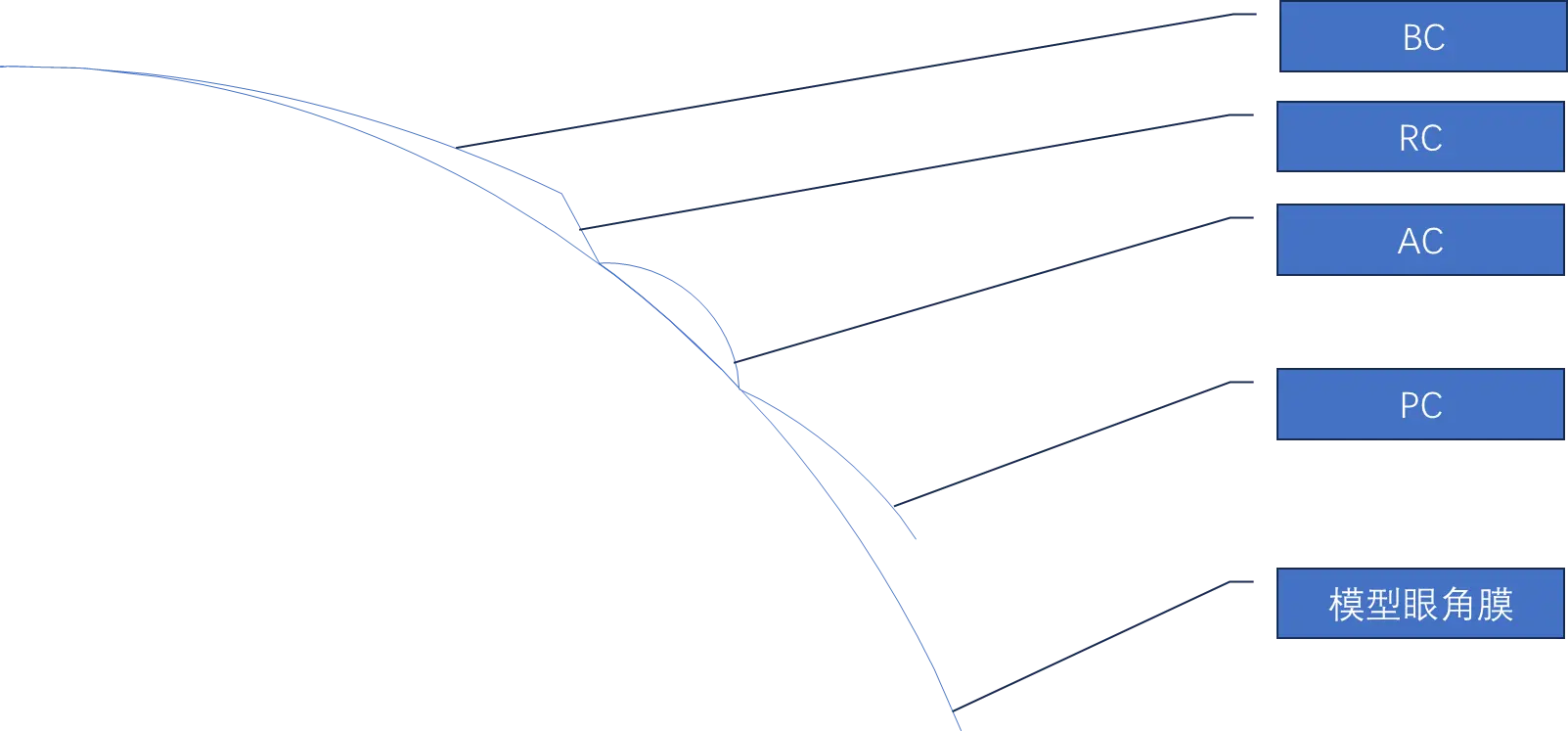
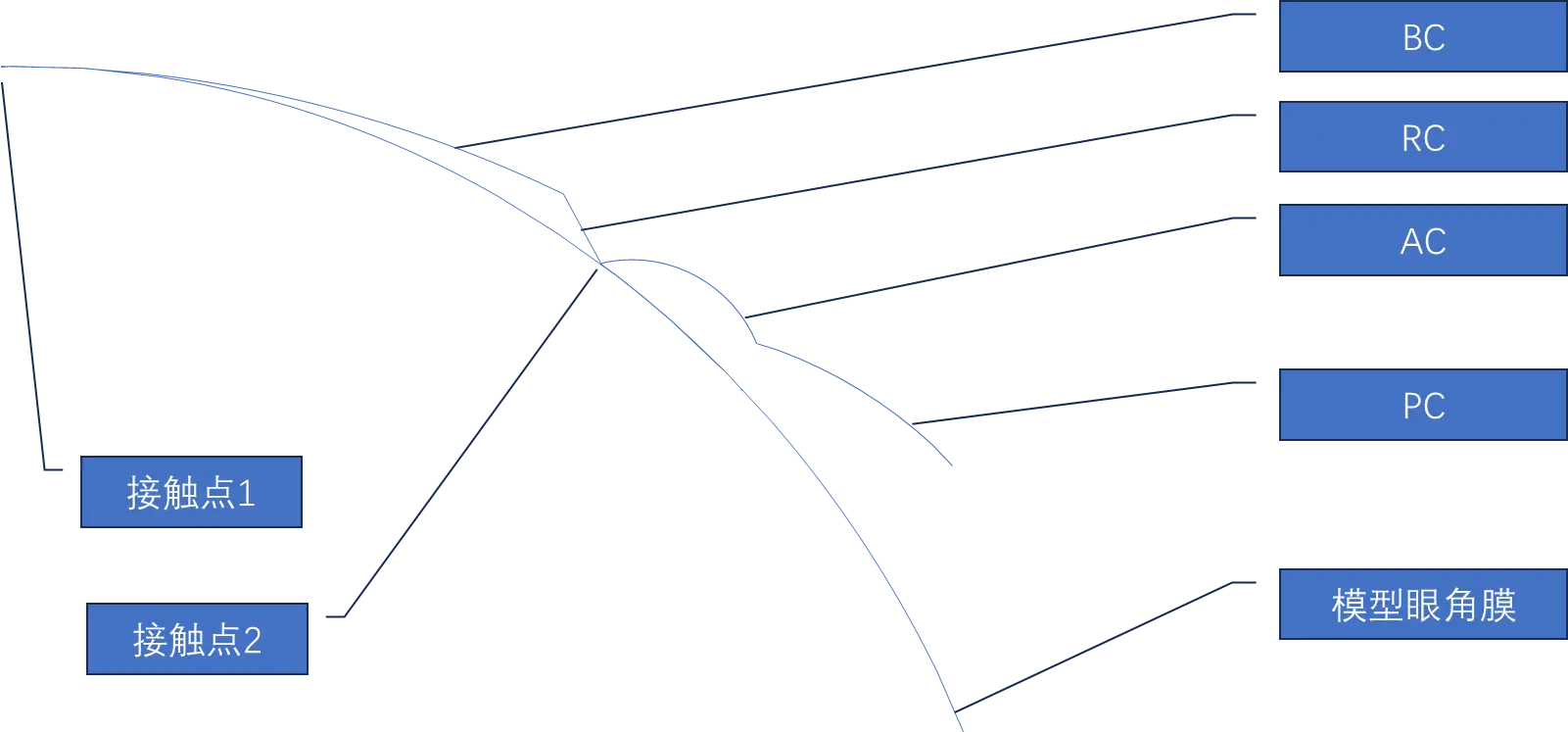
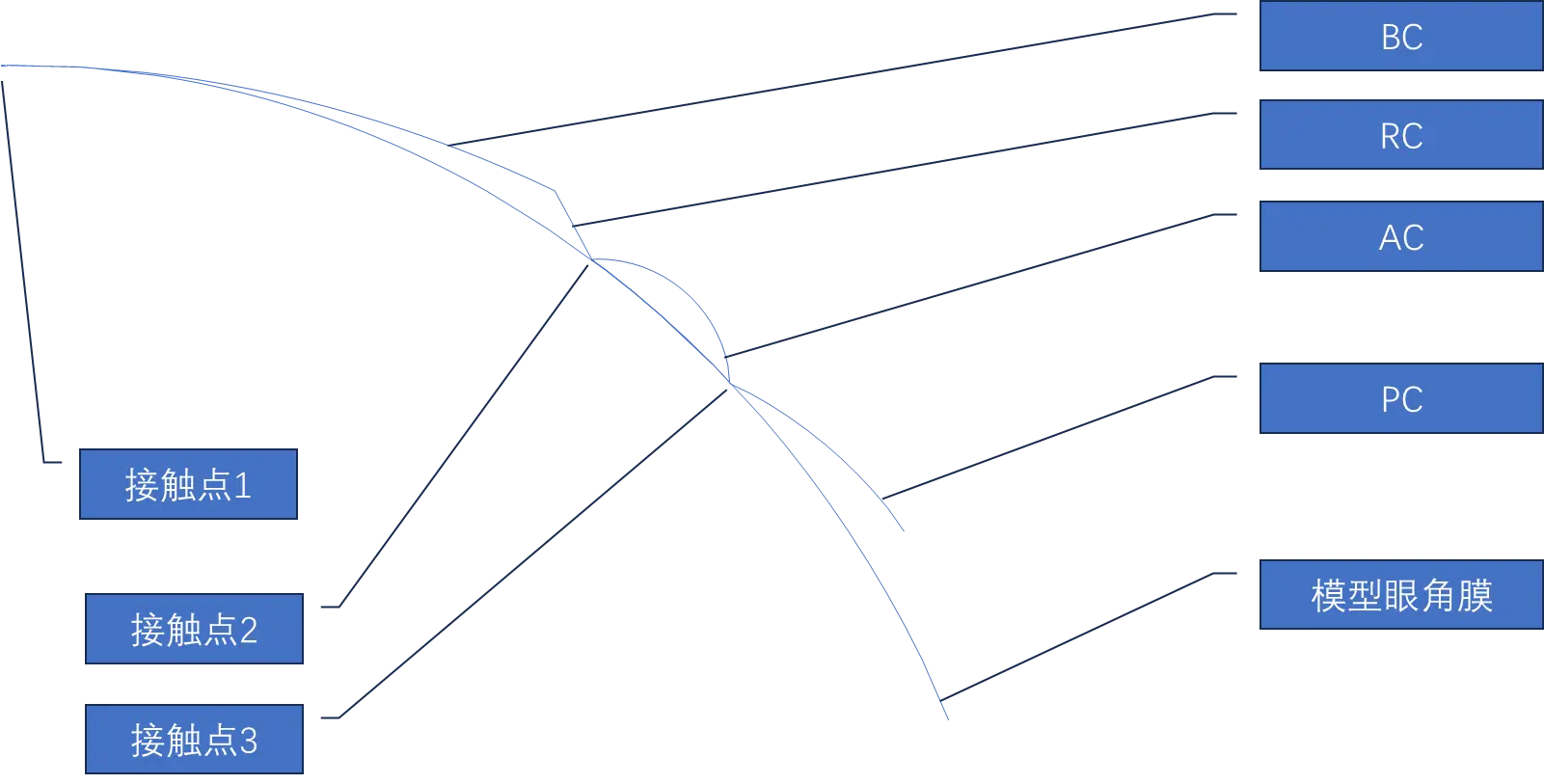
Figure 1 shows a schematic diagram of half of the model eye's cornea and the four arcs of the orthokeratology lens. The AC arc is drawn in an exaggerated manner for illustrative purposes; in reality, it would not be so pronounced. As per our previous agreement, the center of the AC arc is on the lens's axis of symmetry. The radius of the AC arc in the diagram is too small.
According to common ophthalmic models, we assume that the cornea of the model eye is an axisymmetric conical curve shape, determined by two parameters: the radius of curvature R and the conical coefficient Q value (of course, it can also be the e value, but I really, really dislike e).
We previously agreed that this simplest orthokeratology lens has the following structure:
- BC: Circular arc
- RC: Straight line
- AC: Circular arc
- PC: Circular arc
So, the orthokeratology lens and the cornea will have three contact points:
- The starting point of the BC arc, which is the center of the orthokeratology lens. Here, saying "contact" is not rigorous. It is more accurate to say that it is very close, generally needing an orthokeratology lens and corneal center to have a gap of ~5um. But for simplicity, we can first set it as contact, that is, the gap = 0. In order to correct myopia, the shape of the BC arc should be more flat than the cornea. So if the starting point of the BC arc is in contact with the cornea, then the end point of the BC arc must be further away from the cornea and will not have contact again. The end point of the BC arc is the starting point of the RC arc, so the starting point of the RC arc cannot possibly be in contact with the cornea.
- The starting point of the AC arc, the end point of the RC arc is also the starting point of the AC arc. The purpose of the AC arc is to allow the orthokeratology lens to remain stable on the cornea, so the starting point of the AC arc should be in contact with the cornea.
- The end point of the AC arc, should also be in contact with the cornea. Are there other points within the AC arc that can be in contact with the cornea? If you can draw a conical curve (cornea), whether it is a circle, ellipse, parabola or hyperbola, and it can intersect with another circular arc (AC), then on one side of the axis of symmetry, there can be at most two intersections.
(Saying it is a contact point is not rigorous, BC is in contact with the cornea at one point, but the AC arc is circular, so the starting and ending points of the AC arc are two circular lines.)
Can it be less? Maybe, you can choose one of the starting or ending points of the AC arc, but this may not be stable without three contact points. For example, the starting point of the AC arc is in contact with the cornea, and the end point is raised, and there is no contact with the cornea. Is there a possibility of deviation?
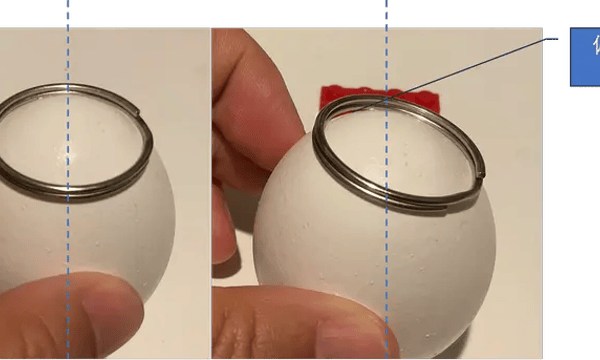
If the asphericity of the cornea is relatively large, for example, please imagine an egg
(Aspheric cornea) and a circular metal key ring (AC arc starting point). If you put the metal key ring on the egg and make it as close as possible to the egg, how many positions can the key ring be stable? Isn't the plane of the key ring perpendicular to the axis of symmetry of the egg?
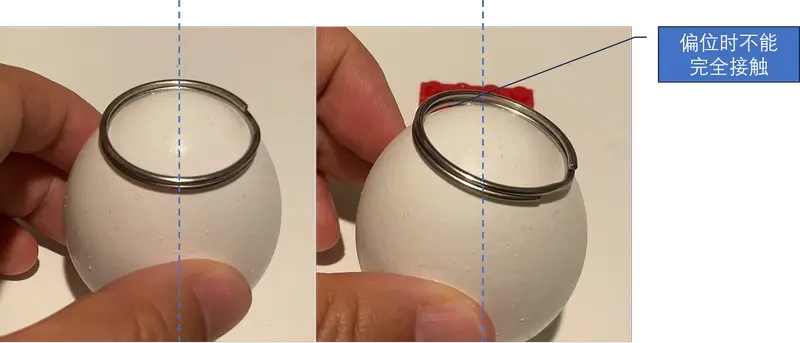
In this tutorial, we choose to use three contact points.
As we will see later, there are as many contact points as there are equations. Similarly, to solve several equations, several types of measurements need to be made on the patient. In most cases, orthokeratology lenses use a three-contact point scheme, requiring three sets of measurements on the patient.
For example: 1. The patient's corneal K value; 2. The patient's optometry; 3. And the on-lens optometry after wearing. Or 1. The patient's corneal K value; 2. The patient's corneal Q value; 3. The patient's optometry.
And the trial lens set of orthokeratology lenses is usually arranged in two dimensions, for example, the horizontal direction may be the K value, or the radius of curvature, and the vertical direction is the "drop degree", that is, the optometry result, so it only provides two variable parameters, which can solve two equations. The solution to the third equation needs to be obtained through on-lens optometry, or through corneal topography.
But if you only use the trial lens of the orthokeratology lens, then there are only 2 variable parameters, and you can solve two equations. If the manufacturer says that's enough, no need for on-lens optometry, then the orthokeratology lens and the cornea, (at least in design), only have two contact points. The third one, it may be in contact, it may not, is an open solution.